I observed an Algebra class recently where students were trying to multiply two polynomials, (x + 5) and (3x2 - 5x - 4). And as I roamed the room, I noticed several students who were stuck because they couldn’t “FOIL it.” Others, meanwhile, did FOIL it and came up with an incorrect product, 3x3 + 15x2 - 4x - 20, as a result (instead of 3x3 + 10x2 - 29x - 20).
The problem, of course, is that the ever-popular FOIL Method only works when multiplying two binomials. Use it when multiplying a binomial by a trinomial, as students did in the above example, and it’s as though the middle term of the trinomial never existed.
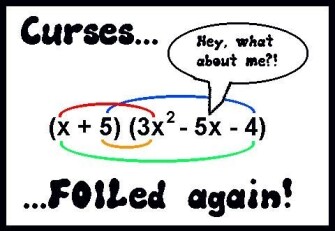
So, how can we prevent such confusion? Simple. Stop introducing FOIL in the first place, as math coach and consultant Michael Goldenberg wrote in response to my earlier post about another misguided math mnemonic, PEMDAS:
And while you’re bumping off Aunt Sally, consider crumpling FOIL as well. That one is so egregiously harmful: not only does it cause at least as much confusion as it allegedly alleviates, but it makes students helpless in the face of other cases of polynomial multiplication. Simply throw a binomial times a trinomial at them and watch them try to apply FOIL. Horrid.
How about it, fellow math educators and curriculum developers? Can we finally put the kibosh on misleading mnemonics like FOIL, and instead stress the math behind a given concept or procedure? In the case of multiplying two polynomials, for example, just show students that it’s another application of the distributive property, where each term in one polynomial is multiplied by each term in the other polynomial.
In other words, let’s forget about catchy but confusing mnemonics, and stick with FOIL-proof methods for explaining mathematics.
Image provided by GECC, LLC with permission