It’s important when demonstrating mathematical procedures to stress the mathematics behind those procedures (i.e., the “why” behind the “what” or “how”), since failing to do so can cause or reinforce students’ misconceptions. Take, for example, multiplying fractions such as 3/5 x 5/8, where teachers commonly--and correctly--note that “the fives cancel out,” leaving 3/8 as the product. But unless students understand why the fives cancel out, they see it as something that happens magically rather than mathematically.
Some students figure they can then wave their magic wands and also cancel out when adding or subtracting fractions--such that the sum of 3/5 and 5/8 would equal the product of 3/5 and 5/8. To prevent errors like this, be sure to explain the mathematical basis for cancelling--i.e., you’re not really cancelling common factors, but rather dividing them to yield a quotient of 1 (represented as 1/1). Then show students--or better yet, have them discover on their own--that while this works when multiplying fractions, it doesn’t work when adding or subtracting them.
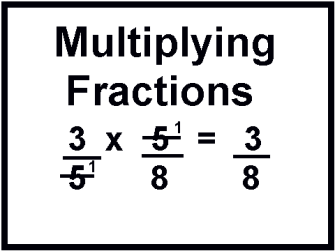
Abracadabra.
Image provided by GECC, with permission.


