This post is by Scott Swaaley (P.E., LEED AP), who teaches ninth grade physics and engineering at High Tech High in San Diego.
As a scientist and engineer, I really like math. The fact that people believe math pedagogy is fundamentally different than other disciplines makes me crazy, and I’m done with the philosophical discussions and the quagmire of research. A few months ago I decided to just try something that feels good and write about it. This is my experiment.
It was February and we were stuck. We were a few months into The Long Now Project, designing a kinetic art piece for a local elementary school, and we were quickly discovering that the physical size of the project required that we have some steel parts fabricated professionally. The first dilemma in formalizing drawings was that the project relies on an esoteric clockwork mechanism called a flying pendulum. As the pendulum swings around, the momentum of the ball deflects the ball at some angle and by some distance G (see Figure below). That distance G is important as it helps to determine if the ball will correctly wrap around the stationary post (see prototype video here). So I went about calculating G.

| This scribbled mess is my first attempt at modeling the system mathematically and was the base of my initial conversations with my colleagues. |
As I worked through the math I quickly realized that there were all sorts of values we needed to optimize. For example, initial calculations showed the pendulum ball moving at a speed of 13 meters / second--30 percent of the speed of an arrow! This is going into an elementary school so that was unacceptable. Just as I started to get overwhelmed by this multivariate optimization problem it hit me--why am I doing this work? This is real world engineering, physics, and math. My 9th graders should be doing this! I want them to understand and solve this problem! It demands a rigorous understanding of physics and math concepts as well as a larger ability to think about and solve a large interconnected system.
I started with my usual plea for help--breakfast with two of our wonderful math teachers. They gave me some great feedback on how to implement this in my classroom. Next, I pitched my refined ideas and plans to my students for feedback. They were surprisingly upbeat about the prospect, even though they were slightly anxious about the complexity of the math. To battle the general fear of complexity for the equations and concepts, I scaffolded it with a series of activities to help alleviate those fears, to practice some of the math, and to become familiar with the overall system.
Students started with a handout that modeled the entire system diagrammatically and algebraically while also defining our independent and dependent variables.
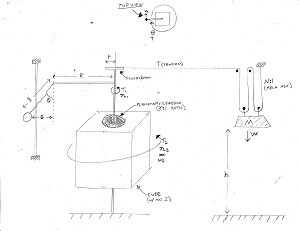
|

|
A diagram of the overall system (full document) | A mathematical model of the system. Dependent variables we were most concerned with are boxed. |
We walked through these models together to normalize our expectations for showing work and tracking units, to practice our algebra, to introduce physics concepts, and also as general tool for developing familiarity with how things are related. Next, students self-selected into specialized physics research groups, went through an independent research process, presented their findings to their peers, and also developed a mathematical intuition about the relationships in the system.

| 
|
Independent Research Organizer | The variable impact chart. It’s basically a process of looking at whether variable are proportional, inversely proportional, or otherwise.
|
At this point they had some of the tools and familiarity they’d need and I know they had been learning to use Desmos, an online graphing tool, in their Math class so I gave them some freedom to explore and think collectively. These were their prompts.

| 
|
Some processes that I thought they might come up with include: guess and check, graphing of variables to help visualize things (perhaps using Desmos), and simplifying all of these individual equations into a single equation. While they impressed me with their self-organization during this process, and the activity fed into our larger conversation about workplace expectations, thinking critically, and grit, they completely missed the boat mathematically. When I asked one student what he thought about this kind of math learning, he expressed sincere frustration because he identified with being “good at math” but was feeling wholly unprepared for applying it. After a long conversation it became clear that they had never had to approach an undefined problem before. When discussing next steps, we decided that the best thing we could do was find a better way to model, visualize, and tinker with the system. We came up with three main ways: spreadsheets, graphing, and algebra.
Then we dove off the deep end. Students self-selected into groups, each responsible for modeling our dilemma in either spreadsheets, multivariate graphing with Desmos, or algebra. This is when I started seeing the returns, the curiosity, and the real learning happening. The number of authentic math discussions we had were beyond measure and each started by an authentic dilemma a student was having. A class conversation strategizing over how best to approach this problem led to a student-driven discussion of recursion and iteration and how most functional math in life is approximation. The discussion of approximation then led to questions about approximating hard shapes (circles) with easy shapes (like squares) which then led into a brief introduction of calculus (rectangles approximating the area under a curve). And this was all instigated by the students!
As they are now in their specific area of mastery, we’ve had all sorts of other mini discussions. When graphs in Desmos became difficult to understand, we talked about asymptotes and limits to infinity. When Desmos misbehaved, we had a discussion on the difference between independent and dependent variables. When students got mired in the algebra, we did short lessons and one-on-one discussions on simplifying fractions over fractions, proper showing of work and process, and inverse and compound trigonometric substitutions. As students dug into spreadsheets, we worked on programmatic representations of equations and LaTeX. It’s been fantastic to watch all of these complex mathematical topics stem from a genuine interest in solving a problem.
Most students finished their models, but in the end, as often happens in life, we didn’t come to a concrete answer. We learned more about the complex relationships and interdependencies, which independent variables made the biggest difference, and which greatly limited the variation in our design, but there were no magic final answers, and the students seemed ok with that.
Example Student Work

|

| These graphs aren’t just pretty pictures. Each plots the dependent variables we deemed most important as a function of different independent variables and each interesting feature could be directly mapped onto a physics concept or a physical limitation of the system itself. Each of these were also animated over a defined range. |
|

| |
This work shows the algebra involved in finding G as a function of only independent variables. |

|

|
Having had a few months to think back about how this went, I have a few final thoughts.
- Project-based math is possible! As in any other discipline, it’s all about creating an authentic context where the mathematics work (and teaching) is in service of a project or other outcome that the students have already bought into. In this case, I could often be heard saying “If we design this wrong, the whole project may fall over onto an elementary student!”
- Several of my colleagues have mentioned that when they give students the choice between “projects” and “problems,” that a vast majority choose the latter. I believe that this is because they’re not bought-in to the project and/or new things are scary and project-based math is hard. Check out my colleague Adam Ko’s master’s thesis on this very question.
- Since the greater Long Now project is not complete yet, we will be going through a refined version of this same process next fall. Fingers crossed. One of our current students is showing our new math teacher how it all works.
- Almost by accident, we hit a huge number of the Common Core Standards, including Quantities, Seem Structure in Expressions, Reasoning with Equations and Inequalities, Interpreting Functions, Building Functions, Modeling, Modeling with Geometry, Geometric Measurement and Dimension, and nearly all of the Mathematical Practices. With a little more forethought, we could easily have hit: Arithmetic with Polynomials and Rational Expressions, Creating Equations, and Trigonometric Functions.
In summary, this experiment was a powerful experience for me. Is the process perfect? Nope. But it’s proving to me that it’s possible!